Math 1800: Multivariate calculus #
Bowdoin College
Functions of more than one variable are ubiquitous: rates of chemical reactions depend on temperature and pressure, crop yields depend on the amount of rainfall and fertilizer used, and most quantities studied in economics depend on dozens of input variables. Multivariate calculus adapts the tools of calculus to this, much richer, setting.
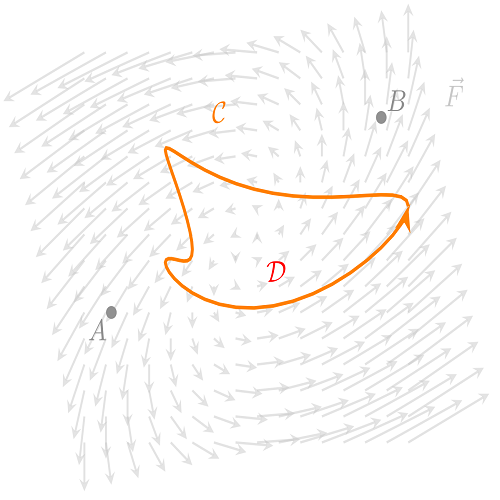
The course starts by developing the language and tools necessary to study multivariate functions, such as graphs, contour diagrams, and vectors. We then develop notions of differentiation and integration as natural analogues of those seen in single-variable calculus. The course ends with the study of new calculus notions unique to the multivariate setting.
Regular coursework is supplemented by laboratory work that introduces students to compelling applications of multivariable calculus and develops intuition for mathematics in higher dimensions.